nextupprevious
Next:About this document ...

BL Lacs in a Complete Sample of Northern RASS Sources


R. Mújica
INAOE-Tonantzintla, Mexico and
Observatoire Astronomique, Strasbourg, France

F.-J. Zickgraf
Observatoire Astronomique, Strasbourg, France

I. Appenzeller, J. Krautter
Landessternwarte-Heidelberg, Germany

A. Serrano
INAOE-Tonantzintla, Mexico

W. Voges
MPE-Garching, Germany
 

Introduction

A defining characteristic of BL Lac objectsis their lack of prominent emission lines, indicative that their radiant energy is largely associated with non-thermal processes. This feature has led to the fact that, BL Lac objectshave been mainly identified for their radio or X-ray emission, with the consequent subdivision of the class into radio-selected BL Lac objects (RBLs) and X-ray-selected BL Lac objects(XBLs). Whether these two classes correspond to different populations or to different aspects of the same phenomenon is still in discussion. In general, they seem to exhibit different properties, with RBLs being more core dominated, highly polarized, variable and luminous at optical and radio wavelength than XBLs (see Perlman & Stocke 1993; Jannuzi et al. 1994; Morris et al. 1991; Padovani 1992). It also seems the two classes occupy different regions in the   diagram, indicative of different energy distributions (Stocke et al. 1985; Ledden & O'Dell 1985).

Extreme variability is among the most striking properties of BL Lac objectsand is therefore well suited as identification criterion. In general BL Lac objectsare variable across the electromagnetic spectrum, although there is a considerable range of variability properties, i.e. amplitudes, timescales, periodicity, etc. (Kollgard 1994).

During the identification program of a sample of ROSAT All-sky Survey X-ray sources in the northern hemisphere (Zickgraf et al. 1997), the intrumentation used did not permit to acquire spectroscopic data good enough to classify the BL Lac objects in a reliable way. It was therefore neccesary to procede in a different way. We monitored all the objects with a suspicious featureless spectrum (from the ID project) and with a 4.85 GHz radio detection. In addition, we computed the diagram for the X-ray/radio sample in order to check the position of the candidates. In this way, a variable object with a suspicious featureless spectrum and adequate spectral indices can be reliable classified as a BL Lac.

Here we present the results about the identification and the analysis of the variability of the BL Lacs, we compare our method with other techniques to identify BL Lacs, and also discuss the results with respect to the unification models.
 
 

Selection of the sample

The original X-ray sample is described in Zickgraf et al. (1997). Six study areas north of were investigated. This complete, flux and area limited sample consists of 674 X-ray sources.

Based on the fact that there are no radio quiet BL Lac objects(Stocke et al. 1990), we crosscorrelated the sample with radio catalogues. We have selected those X-ray sources with a counterpart in the 87GB catalogue (Gregory & Condon 1991) or in the BWE Catalogue (Becker, White & Edwards 1991), both at a frequency of 4.85 GHz.

As the position uncertainty of both catalogues is , we selected only the X-ray sources with a radio counterpart located closer than 120" from the X-ray position. From this correlation we obtained 67 sources. This is the X-ray/radio sample. We selected the objects with a suspicious featureless spectrum and started to monitored them for optical variability.
 
 

Observations and Data Reduction

The observations were taken during seven runs with the 2.1m telescope of the Guillermo Haro Observatory in Cananea, Sonora, Mexico, and in the 1.23m telescope of the Calar Alto Observatory in Spain.

The data on the 2.1m telescope were collected with the Landessternwarte Faint Object Spectrograph and Camera (LFOSC). This instrument has a focal reducer that permits to obtain photometry and multi-object spectroscopy. It has a CCD with 22m pixel size as a detector and the field size is 10' X 6' (the scale is 1"/pix) (see Zickgraf et al. 1997). The 1.23m telescope was equipped with a TEK CCD and a Rc filter. The field size was 10' X 10'.

Each of the fields was observed, at least, once per night. The frames were reduced following a standard procedure (bias substraction, flat-field correction). In order carry out the differential photometry, we simulated aperture photometry in our frames. In addition to the BL Lac(s) candidate(s), we selected, at least, five non-saturated comparison stars of different brightness. The errors were estimated from the comparison stars with same brightness as the candidates.

We computed the ratios of the countrates for each pair of objects and then we normalized them over all the data available. The variable comparison objects were rejected from the analysis. The final light curves were computed using one of the non-variable comparison stars as bright as the candidate and the check star was as bright or brighter than both (candidate and comparison). The errors are typically 1-3% and in the worst cases 5%. An example of a light curve is plotted in Figure 1.

For the final list of candidates, additional data were obtained with LFOSC (dispersion 360Å mm-1) in order to improved the S/N ratio. As an example, the spectrum of one object (same as the light curve) is shown in Figure 2.
 
 

 
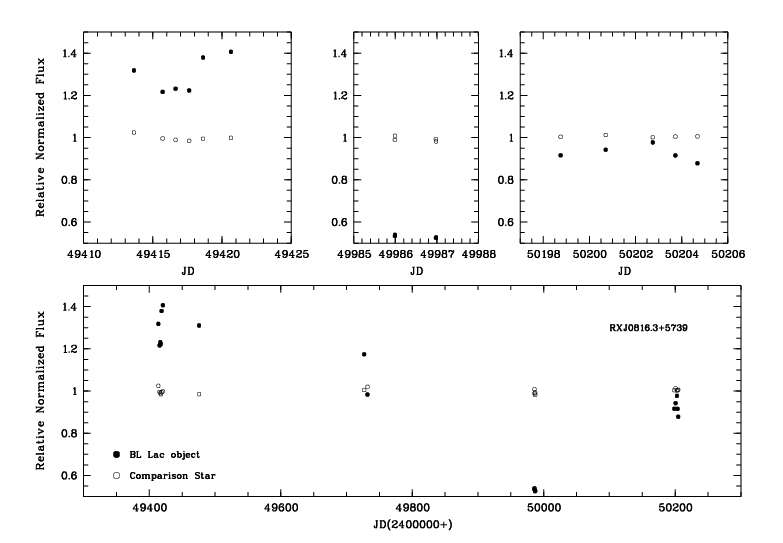
Figure 1: Light curve of RXJ0816.3+5739 (B179).
 

Figure 2: Spectrum of RXJ0816.3+5739 (B179).

Variability Statistics and Amplitudes

Test

In order to measure the significance of the variability of the objects in the sample, we applied the test to the light curves (Penston & Cannon 1970). We compute the probability P of the function and consider an object as variable, if it has a probability  P > 99.5 %  (Heidt & Wagner 1996). The results are given in Table 1.
 
 
 

Table 1: Results from the Test and Amplitudes.
NAME ROSAT Name P(%) Amp(%) Amin Amax sigma Notes
A073 RXJ0349.9+0640 192.74 >99.7 13.9 0.941 1.080 0.010  
A158 RXJ0416.8+0105 199.81 >99.7 33.8 0.785 1.174 0.027  
B027 RXJ0710.4+5908 239.30 >99.7 15.3 0.903 1.057 0.013  
B158 RXJ0806.4+5931 665.55 >99.7 20.7 0.937 1.144 0.009  
B179 RXJ0816.3+5739 11674.80 >99.7 88.1 0.525 1.406 0.012  
B181 RXJ0816.6+6208 361.19 >99.7 52.6 0.598 1.125 0.024  
D096 RXJ1217.8+3007 776.20 >99.7 51.3 0.767 1.281 0.023  
D113 RXJ1221.3+3010 60.55 >99.7 12.4 0.931 1.056 0.016  
D115 RXJ1221.5+2813 1822.79 >99.7 78.5 0.651 1.436 0.021  
E089 RXJ1704.8+7138 415.75 >99.7 37.1 0.761 1.134 0.022  
E211a RXJ1732.0+6926 16.50 98.8 42.3 0.784 1.230 0.100 Faint
E211b RXJ1732.0+6926 11.52 92.6 9.1 0.938 1.036 0.024 Bright
F102 RXJ2233.0+1335 317.60 >99.7 33.2 0.912 1.245 0.018  

Amplitudes

We computed the amplitudes in order to check if there exists a correlation with other properties of the BL Lacs. These were calculated following Heidt & Wagner (1996):

where Amax is the maximum value of the light curve, Amin is the minimum and is the one sigma error derived from the comparison stars. Table 1 contains the values of these quantities.
 
 

Discussion

Variability

We found that nearly all selected BL Lacs are variable. Only one of the selected objects did not show significant variability. The timescale of the variations is different from object to object. There are cases for which the variations were easily identified already on timescales of days, in others on timescales of months. However, some of the objects only showed variations until we used archived data from the identification program, which extended the covered timescales to a couple of years. In Table 2 we have summarized the timescales of the variations shown by each object.
 
 
 

Table 2:  Variability timescales present in the BL Lac light curves. Days is for timescales less than a week, week for timescales less than a month and so on. Y means there exist variability on the timescale of the column;  Y: means there is some evidence but variation is not clear; X means there is no variation on that timescales; - is for the timescales we do not have data.
Nov93 ROSAT Name days weeks months years
A073 RXJ0349.9+0640   Y    Y      Y X
A158 RXJ0416.8+0105 Y: Y:      Y    Y
B027 RXJ0710.4+5908 Y: - - -
B158 RXJ0806.4+5931   Y X      Y    Y
B179 RXJ0816.3+5739   Y     Y      Y    Y
B181 RXJ0816.6+6208   Y  Y: Y: Y:
D096 RXJ1217.8+3007   Y -      Y -
D113 RXJ1221.3+3010 Y: -      Y -
D115 RXJ1221.5+2813   Y     Y      Y -
E089 RXJ1704.8+7138   Y     Y      Y    Y
E211a RXJ1732.0+6926   Y -      Y -
E211b RXJ1732.0+6926 X - X -
F102 RXJ2233.0+1335 Y:     Y      Y     Y

Note that the objects which show questionable variability (:) on the shorter timescales we measured (days), are the ones which are X-ray brighter (see Table 3). This fact is also reflected in the  diagram (Figure 3) where these objects are located more to the left in the diagram. Therefore, X-ray brighter BL Lacs seem to be less variable on shorter timescales (of the order of days) than X-ray faint ones.

Diagram

Two point spectral broad band indices ( diagram) have been extensively used in the last years for the classification of extragalactic objects (Stocke et al. 1991) and for the discovery of new BL Lac objects (Schachter et al. 1993), because most of the identified XBLs ocuppy a unique area in the radio/optical/X-ray (or -) color-color diagram, compared to other extragalactic classes.

The effective spectral indices between two bands i and j are defined as:

where Si and Sj are the monochromatic fluxes at the frequencies and , respectively (Tananbaum et al. 1979; Stocke et al. 1985; Ledden and O'Dell 1985).

For the optical-to-X-ray () and radio-to-optical () the monochromatic fluxes correspond to 2keV for X-ray, 2500Å for optical and 4.85 GHz for radio bands (Stocke et al. 1991).

In order to calculate the total X-ray flux fx in the PSPC band (0.1-2.4keV), we used the galactic hydrogen column density from Dickey & Lockman (1990) as computed for each source individually by using the online facilities in EXSAS. Then, we calculated the total energy flux assuming a photon index . Finally, the flux at 2keV was calculated assuming a power law flux distribution with .

Optical magnitudes were obtained from the digitized sky surveys plates. These were tranformed to fluxes at 2500Å following Schmidt (1968). All the monochromatic fluxes were K-corrected for the redshift of the object, assuming   and . For those objects without a measured redshift, we adopted the value z=0.3 equal to the mean redshift value of the BL Lacs with measured redshift contained in the EMSS (Stocke et al. 1991). Table 3 contains the optical, radio and X-ray data for our sample.
 
 
 

Table 3: Data for the Sample. The meaning of the columns is as follows: (1)Control name; (2) ROSAT name; (3) ROSAT countrate (cts s-1); (4) ROSAT total flux (ergs cm-2 s-1); (5) Column density (1021cm-2); (6) E (red) magnitude from the digitized plates; (7) O (blue) magnitude from the digitized plates; (8) Redshift; (9) Absolute magnitude; (10) Radio flux at 4.85Ghz (mJy); (11) Radio spectral index from BWE; (12) X-ray flux at 2kev (mJy); (13) Optical flux at 2500Å (mJy); (14) ; (15) ; (16) logfx/fv; (17) Notes
nov93 NAME countrate fx NH E O z Mabs
(1) (2) (3) (4) (5) (6) (7) (8) (9)
A073 RXJ0349.9+0640 0.061 2.137e-12 1.249 18.45 19.56 0.300 -21.43
A158 RXJ0416.8+0105 1.720 5.656e-11 1.046 16.08 17.10 0.287 -23.78
B027 RXJ0710.4+5908 1.000 2.608e-11 0.574 15.40 17.36 0.125 -21.61
B158 RXJ0806.4+5931 0.188 4.224e-12 0.414 16.27 17.16 0.300 -23.83
B179 RXJ0816.3+5739 0.104 2.437e-12 0.452 16.87 17.81 0.300 -23.18
B181 RXJ0816.6+6208 0.031 7.097e-13 0.419 18.35 18.78 0.300 -22.21
D096 RXJ1217.8+3007 2.830 3.998e-11 0.171 13.86 15.50 0.237 -24.94
D113 RXJ1221.3+3010 1.600 2.274e-11 0.173 15.71 16.50 0.130 -22.56
D115 RXJ1221.5+2813 0.157 2.331e-12 0.188 13.69 16.50 0.102 -22.01
E089 RXJ1704.8+7138 0.095 2.145e-12 0.414 15.80 16.53 0.300 -24.46
E211a RXJ1732.0+6926 0.013 2.975e-13 0.398 * 20.47 0.300 -20.52
E211b RXJ1732.0+6926 0.013 2.975e-13 0.398 * 19.04 0.300 -21.95
F102 RXJ2233.0+1335 0.108 2.613e-12 0.484 * 19.40 0.300 -21.59
nov93 f4.85 f2kev f2500 logfx/fv Notes
  (10) (11) (12) (13) (14) (15) (16) (17)
A073 73 -0.70 1.392e-04 1.913e-02 0.82 0.47 1.72  
A158 70 * 3.682e-03 1.849e-01 0.65 0.47 2.16 H0414+009
B027 81 -0.40 1.698e-03 1.516e-01 0.75 0.50 1.93 EXO0706.1+5913
B158 42 * 2.750e-04 1.745e-01 1.08 0.43 1.06  
B179 68 * 1.587e-04 9.589e-02 1.07 0.52 1.08  
B181 34 * 4.620e-05 3.925e-02 1.12 0.54 0.93  
D096 445 -0.20 2.603e-03 8.171e-01 0.96 0.50 1.37 ON325
D113 97 * 1.480e-03 3.342e-01 0.90 0.45 1.52 2A1219+305
D115 981 -0.20 1.517e-04 3.368e-01 1.28 0.64 0.54 ON231
E089 43 * 1.397e-04 3.117e-01 1.28 0.39 0.51 Nass 1996
E211a 27 -0.80 1.937e-05 8.276e-03 1.01 0.65 1.23  
E211b 27 -0.80 1.937e-05 3.089e-02 1.23 0.54 0.66  
F102 33 * 1.701e-04 2.217e-02 0.81 0.58 1.75  

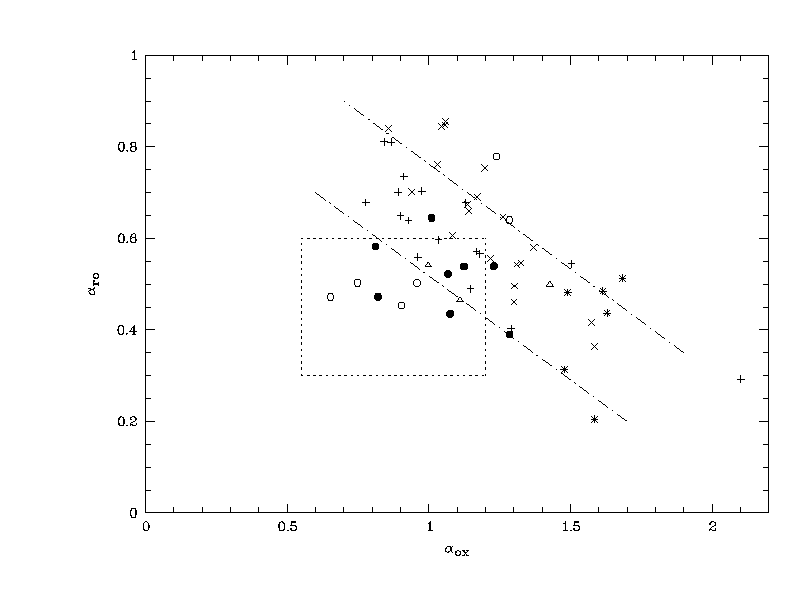
Figure 3 shows the  diagramfor our sample. The different types of objects are represented by the different symbols explained in the figure caption. The dashed square represents the limits given by Schachter et al. (1993) for the region occupied by the XBLs. The dash-dotted lines are the limits for the regions occupied by XBLs (lower-left) and RBLs (upper-right) according to Padovani & Giommi (1995).

The most important thing to notice from the diagram is the position of the known BL Lacs and the new ones. The known objects fall in the distinct regions of the diagram described above, as if there were two separated groups, XBLs and RBLs.

Based on this fact, several authors (Garilli et al. 1990; Stocke et al. 1991) have claimed that the population of BL Lacs is divided into two groups: XBLs and RBLs. The regions in the  diagram occupied by both groups are displayed in Figure 3. From our diagram it is clear that there are BL Lacs which ``fill'' the gap between these two BL Lac classes (see also Nass et al. 1996; Kock et al. 1996). Whereas the known BL Lacs form two separate groups in the diagram, the RBLs and the XBLs, the gap disappears if the new BL Lacs from our survey are included. It is possible that the bimodality is artificially introduced by the properties of the samples used. For instance, the BL Lacs from the EMSS tend to almost exclusively populate the XBL-region of the diagram. This can be a consequence of the follow-up identification programs that have concentrated on objects inside the square (Schachter et al. 1993; Perlman et al. 1996).

Our data reveal the existence of an intermediate type of BL Lacs. These objects are located between RBLs and XBLs in the  diagram. Kock et al. (1996), Nass et al. (1996) and more recently Laurent-Muehleisen et al. (1997) also found several of these BL Lacs in their samples. This finding has been interpreted as a transition in the broad band properties from one class of BL Lacs to the other and put some restrictions to the unification models.

For instance, Padovani and Giommi (1995) proposed that RBLs and XBLs have the same range in orientation angles with respect to the line of sight, but intrinsically different spectral energy distributions (SEDs) and suggested that the main difference between XBLs and RBLS is the the frequency at which the synchrotron break occurs (Different Energy Cutoff scenario). This model predicts the existence of two separated groups of BL Lacs in the  diagram. Padovani and Giommi were able to reproduce the distribution of the EMSS and the 1 Jy samples accurately in this diagram. However, the existence of intermediate objects was not taken into account in this model.

On the other hand, in the different viewing angle model, the properties of the objects depend on the orientation of the jet with respect to the vieving angle. The RBLs are the objects with smaller angles, where the stronger radio emission rises more steeply, i.e., the spectral indices shift toward the upper right corner in the  diagram.

If the class of BL Lac is determined only by the orientation, it is expected a smooth variation of the properties with varying viewing angle relative to the jet axis. However, there are other properties of BL Lacs that can not be explained only as a geometrical effect (Sambruna el al. 1996; Heidt & Wagner 1998). Nevertheless, a model based in a varying viewing angle and the kinetic luminosity of the jet (Georganopoulos & Marscher, 1998) predicts the continuos distribution of BL Lacs in the  diagram among other properties.

In this way, it is possible to say that the BL Lacs form one group of objects that was separated in two groups due to selection strategies. This is also supported by the continuous variation of amplitudes with the spectral indices.
 
 

 
Figure 3:  diagram for our sample. Filled circles represent the new BL Lacs, open circles the known ones, plus symbols are Seyfert Galaxies, crosses correspond to QSOs, triangles are galaxies in a cluster and asterisks are normal galaxies.

Correlations

We plotted the amplitude of the light curves versus the spectral indices of the BL Lacs in order to check if there exist a correlation between the energy distribution and the variability. We found a dependence of the amplitude on the three indices, , and . The correlation of amplitude and spectral index is strongest for , where objects with flatter overall spectrum, from radio to X-ray, show smaller amplitude in the light curve.

For   there is also a clear correlation whereas for  it is less significant. For this index an inclined upper envelope of the amplitude-spectral index distribution seems to exist. These correlations suggest a continuous transition from XBLs to RBLs.

It is possible that for some of the objects, with only a couple of runs, we are not measuring the highest amplitude. Longer time coverage could improve the determination of the amplitude.
 
 

 
Figure: a. Correlation of Amplitude vs .
 

Figure: b. Correlation of Amplitude vs .

Figure: c. Correlation of Amplitude vs .

Comparison of Search Techniques

The most common methods employed to select BL Lacs from a sample of X-ray sources are based mainly on the values on the ratios of monochromatic fluxes in the optical, X-ray and radio band.

The  diagram has proven to be a very useful tool to classify extragalactic objects (Stocke et al. 1991), especially to discover new BL Lac objects (Schachter et al. 1993). However, for some intermediate BL Lacs it is not the best way to identify them since a search restricting candidate selection to the XBL and RBL regions would find XBLs or RBLs. This diagram is very useful, but needs to be complemented with other properties of the BL Lacs such as polarization or variability.

Stocke et al. (1985) also proposed to use the  log(fx/fv)(= logfx + V/2.5 + 5.37) to discriminate BL Lacs from other X-ray emitters since BL Lacs are those AGN which show the highest values of the fluxes ratio (corresponding to the lowest ). Selecting candidates with high log(fx/fv) has the advantage that it is independent of radio observations. Nass et al. (1996) found that 40% of the objects showing log(fx/fv) > 1.3were confirmed BL Lacs via spectroscopy.

We computed the log(fx/fv) ratios for the objects in our sample. The histogram in Figure 5 shows the distribution of the whole sample of radio sources. The distribution of the BL Lac objects is represented by the shaded area.
 
 

 
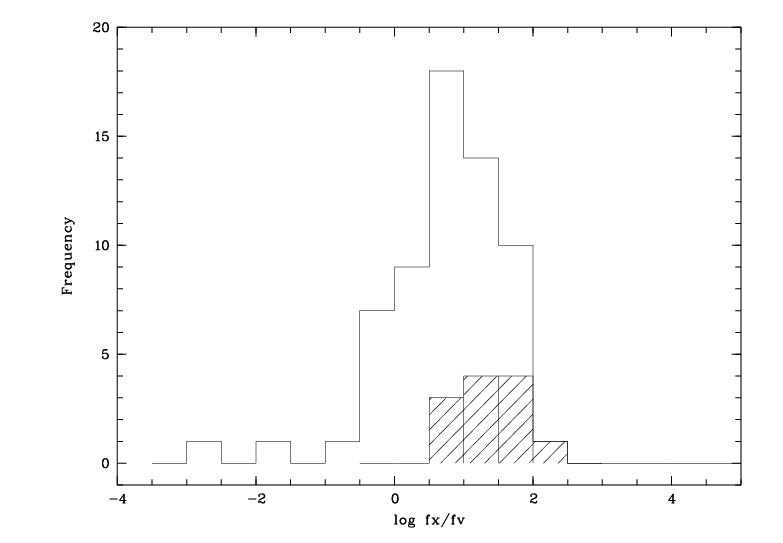
Figure 5: Distribution of the X-ray/radio sample (empty) and BL Lacs (shaded).

It can be seen from the figure that for those objects with log(fx/fv) > 1.5, aproximately 50% are, in fact, BL Lacs. However, worth noticing is that the rest or 50% of the BL Lacs have  log(fx/fv) < 1.5. These objects would be ignored by using exclusively this criteria. This situation is similar as in the case of the  diagram. All of these objects correspond to the intermediate population discussed in the  diagram of our sample.

Summarizing, these two techniques seem to be highly efficient to pre-identify BL Lacs from large samples. However, if the goal is to identify all the BL Lacs in a sample, in order to test unification models, it is better to used complementary criteria.
 

Conclusions

We tested the optical variability as an extra tool to identified BL Lacs in a sample of X-ray sources. We demonstrated that variability studies are helpful to identify BL Lac objects.

For the identification we also used as another tool their distinctive X-ray/optical/radio colors ( diagram). However, an important result is that part of the BL Lacs in our sample show properties intermediate between RBLs and XBLs, which could make their identification, based only in their position in the diagram, more difficult.

It seems that the optical variability is related with the broad-band properties. We found a correlation between the amplitude and the spectral indices. In addition, we have found that the timescales of the variations are shorter for RBLs than for XBLs. This can be seen from Table 2 where the objects with smaller  are suspicious to be non-variable on the timescales of days, while the rest are clearly variable in this timescale.

The correlations in addition to the existence of intermediate objects, favor the idea that BL Lacs form only one population of objects that has been separated in two subgroups due to the strategies followed for preselection of candidates.

The existence of intermediate values in the spectral indices exhibited by BL Lacs in our sample has important implications for unification models. A recent model, based in the orientation angle of the relativistic jet and the kinetic luminosity of the jet, predicts the existence of the intermediate objects.

The techniques discussed in the last section (logfx/fv and  diagram) seem to be highly efficient to pre-identify BL Lacs from large samples. However, as we discussed, not all the objects are picked with this technique. There are several BL Lacs that could have been missed, and if what we desire is to identify all the BL Lacs in a sample (in order to achieve completeness), the candidates cannot be chosen only based in their SEDs. It would be better to make use of as many properties as possible to recognize these objects.
 
 
 

References
nextupprevious
Next:About this document ...
Raul Mujica

1999-01-29